02-12-2024 - Analytic Geometry - Quadrics [EN]-[IT]
hive-146620·@stefano.massari·
0.000 HBD02-12-2024 - Analytic Geometry - Quadrics [EN]-[IT]
 --- *~~~ La versione in italiano inizia subito dopo la versione in inglese ~~~* --- **ENGLISH** **02-12-2024 - Analytic Geometry - Quadrics [EN]-[IT]** With this post I would like to give a brief instruction about the topic mentioned in the subject (code notes: X_53) ***Quadrics*** **What are they** Quadrics are algebraic surfaces of degree two and almost all the ideas about conics can be generalized to quadrics. **Definition** A quadratic is an algebraic surface of degree 2, that is, it is the set of points that satisfy a polynomial equation p(x,y,z)=0 of degree 2. For example, spheres, cones and cylinders are quadratics of degree 2. A pair of planes is a quadratic. In fact, its equation 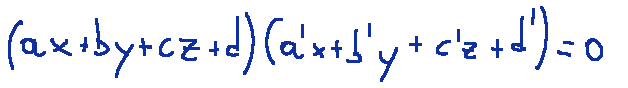 is a polynomial equation of degree 2. **Observation** The homogeneous equation of a quadratic can be thought of as a quadratic form. The matrix associated with it is a 4 x 4 symmetric matrix A such that 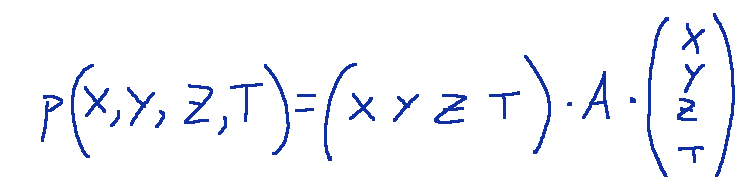 **Further definition** Given a quadratic S with homogeneous equation 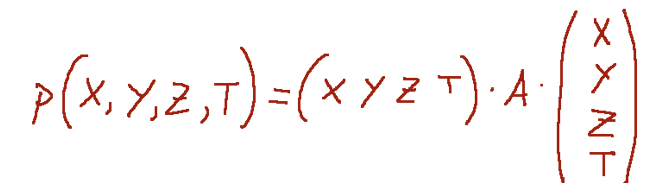 with the notation of the previous observation, the matrix A is called matrix associated with the quadratic S. **Degenerate Quadratics** A quadratic with associated matrix A is said to be degenerate if the determinant of A is equal to 0. 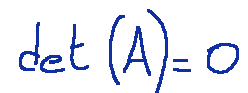 **Euclidean classification of quadrics** A non-degenerate quadric S with associated matrix A can be represented by one of the following Euclidean canonical forms with an appropriate choice of the Cartesian reference system. Below are their algebraic representations. -real ellipsoid  -imaginary ellipsoid 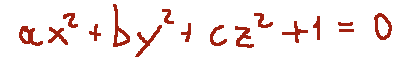 -hyperboloid elliptical  -hyperbolic hyperboloid 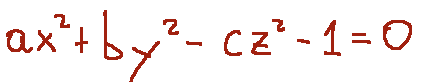 -paraboloid elliptic 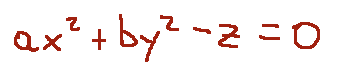 -hyperbolic paraboloid 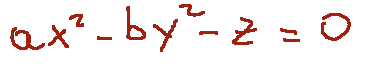 ***Conclusions*** In analytical geometry, quadrics are second-degree algebraic surfaces in three-dimensional space (ℝ³), described by a general equation written as follows: 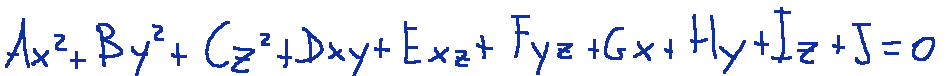 ***Question*** In my opinion, quadrics are a little-known topic in the study world and are a fairly complex part of analytical geometry, do you also think so or not? --- https://images.hive.blog/1536x0/https://files.peakd.com/file/peakd-hive/green77/gGQutTRs-hive-spacer.png --- **[ITALIAN]** **02-12-2024 - Geometria analitica - Quadriche [EN]-[IT]** Con questo post vorrei dare una breve istruzione a riguardo dell’argomento citato in oggetto (code notes: X_53) ***Le quadriche*** **Cosa sono** le quadriche sono superfici algebriche di grado due e quasi tutte le idee sulle coniche possono essere generalizzate alle quadriche. **Definizione** Una quadratica è una superficie algebrica di grado 2, ossia è l’insieme dei punti che soddisfano un’equazione polinomiale p(x,y,z)=0 di grado 2. Ad esempio le sfere, i coni ed i cilindri sono quadratiche di grado 2. Una coppia di piani è una quadratica. Infatti la sua equazione 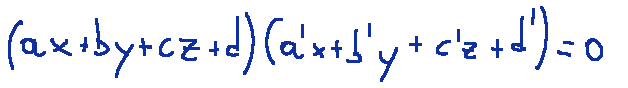 è un'equazione polinomiale di grado 2. **Osservazione** L’equazione omogenea di una quadratica può essere pensata come una forma quadratica. La matrice associata ad essa è una matrice 4 x 4 simmetrica A tale che 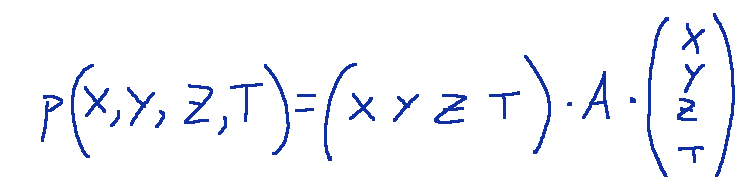 **Definizione ulteriore** Data una quadratica S con equazione omogenea 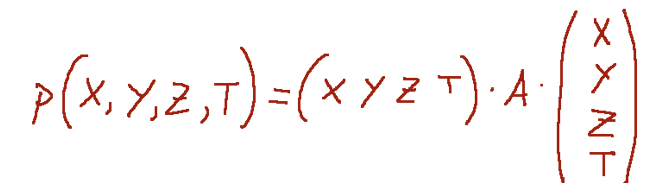 con la notazione della precedente osservazione, la matrice A è detta matrice associata alla quadratica S. **Quadratiche degeneri** Una quadratica con matrice associata A è detta degenere se il determinante di A è uguale a 0. 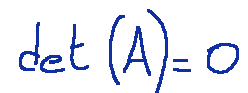 **Classificazione euclidea delle quadriche** Una quadrica non degenere S con matrice associata A può essere rappresentata da una delle seguenti forme canoniche euclidee con una scelta opportuna del sistema di riferimento cartesiano. Qui di seguito le loro rappresentazioni algebriche. -ellissoide reale  -ellissoide immaginario 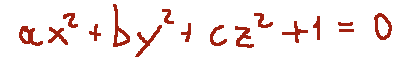 -iperboloide ellittico  -iperboloide iperbolico 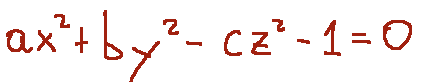 -paraboloide ellittico 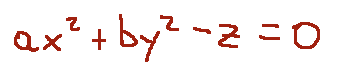 -paraboloide iperbolico 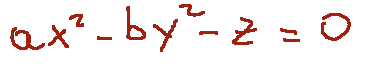 ***Conclusioni*** In geometria analitica, le quadriche sono superfici algebriche di secondo grado nello spazio tridimensionale (ℝ³), descritte da un'equazione generale scritta come segue: 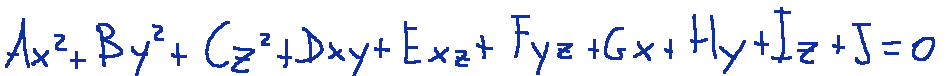 ***Domanda*** Secondo me, le quadriche sono un argomento poco diffuso nel mondo dello studio e sono una parte della geometria analitica abbastanza complessa, anche secondo voi è così oppure no? **THE END**
👍 pab.ink, dexpartacus, vimukthi, healthymary, magic.ctp, hardrockcrypto, magic.vyb, theshot2414, lolz.ctp, marianaemilia, dosh, bastter, goliathus, adulruna, hkinuvaime, stefanialexis, shaidon, gabrielrr17, pardinus, pedrocanella, gomster, caaio, anjanida, seuamiguto, carlosro, brucolac, qyses, kojiri, lobaobh, jpleron, elfino28, highfist, canellov, carl05, deggial, dudeontheweb, garlet, voter, spurisna, sportgamer, libertypal27, libertypob27, axel-blaze, meppij, sweetunicorn, cur8, odb-contest, nainaztengra, libertycrypto27, cryptomonica, ilcontabile, clifth, pixresteemer, sanjeev021, mesk, dragonmk47, khushboo108, pensieristupendi, skiptvads, libertyleo27, bencwarmer, fedesox, stea90, lorenzopistolesi, listnerds, feanorgu, abilitysend, peeyush, ctptips, steemitboard, marivic10, he-index, elianaicgomes, peerfinance, arcange, steemtelly, smartvote, afterglow, shainemata, laruche, walterjay, aidefr, robotics101, studio666, twoitguys, ykretz, flewsplash, sbi4, sbi-tokens, roozeec, lartist-zen, irivers, uyoho, lara77, lee1938, giuatt07, cryptoyzzy, hive-129556, tosolini, sterex, hivebuzz, lizanomadsoul, manncpt, jnmarteau, crypticat, pinmapple, roelandp, discovereurovelo, fronttowardenemy, xsasj, itchyfeetdonica, princessmewmew, cranium, musicandreview, kimzwarch, gabrielatravels, kaminchan, holovision.stem,