Hi there. In this math guide, I cover the distributive law from high school algebra. It is assumed that the reader is familiar with working with negative numbers, multiplication and combining like terms.


<center><img src="https://cdn.pixabay.com/photo/2015/11/15/07/44/learn-1044076_960_720.jpg" /></center>
<center><a href="https://cdn.pixabay.com/photo/2015/11/15/07/44/learn-1044076_960_720.jpg">Pixabay Image Source</a></center>


&nbsp;

## Viewing Distributive Law As An Area Of Rectangle
---

Before getting right into the examples and the algebra I wanted to display the distributive law as an area of a rectangle. This provides a better insight on what the distributive law is doing when working with the algebra. The area of a rectangle is length multiplied by width. If the length and the width are known the area can be determined. If one or both of the length and width are not known we need algebra tools to complete the multiplication. The distributive law is helpful if one of the length or width is known and the other is not known.

<center>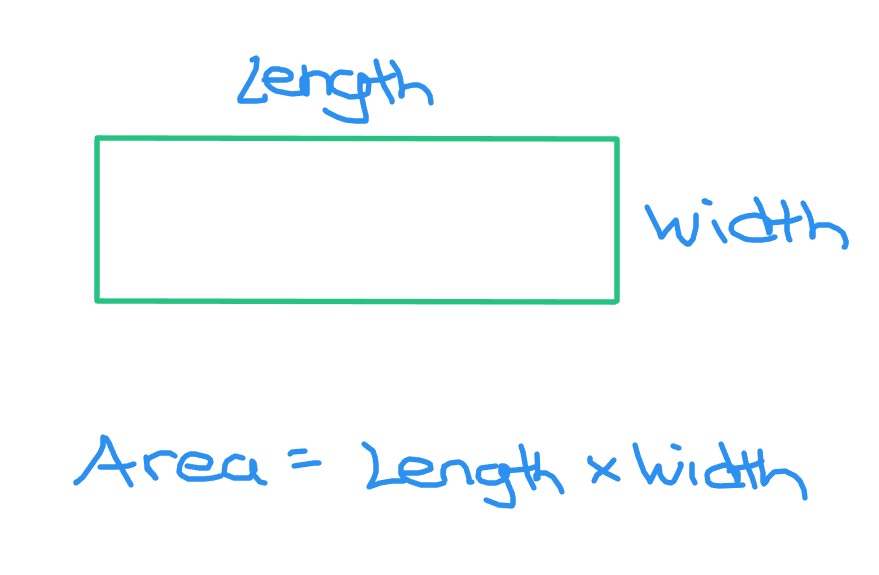
![areaRectangle.PNG](https://images.hive.blog/DQmVECvUjHELsEGdzPxEdYfyt4PZt3CNxFYjwbpDdXagEGx/areaRectangle.PNG)
</center>

This area of a rectangle model is not great if you multiply by a negative number. 


&nbsp;

## Examples
---

Here are some distributive law examples with picture screenshots.

**Example One**

What is `7(x + 3)`?

Multiply 7 from outside the bracket with the `x` in the bracket. This gives `7x`. Next  multiply the seven from the outside with the 3 inside the bracket. This gives 21. The result is `7x + 21`.

<center>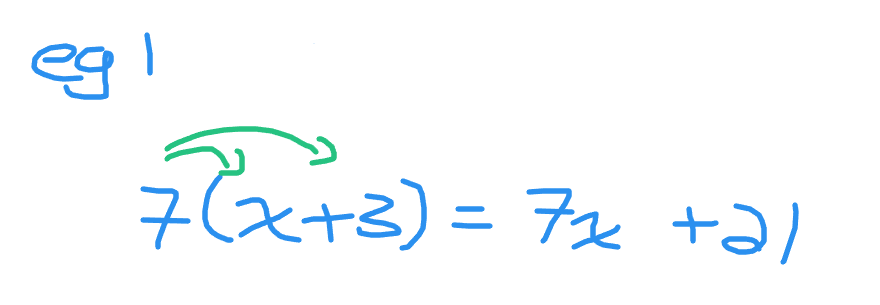
![example01.PNG](https://images.hive.blog/DQmVQX3hFcMvceP6pLK8L6UrTF6LSBdyYk7nzdNMGmarejq/example01.PNG)
</center>

&nbsp;

**Example Two**

What is `10(2x + y - 3)`?

Multiply the ten from the outside of the bracket with `2x` then add `10 times y`. Watch the negative when multiplying ten with negative 3 to get negative 30. 

<center>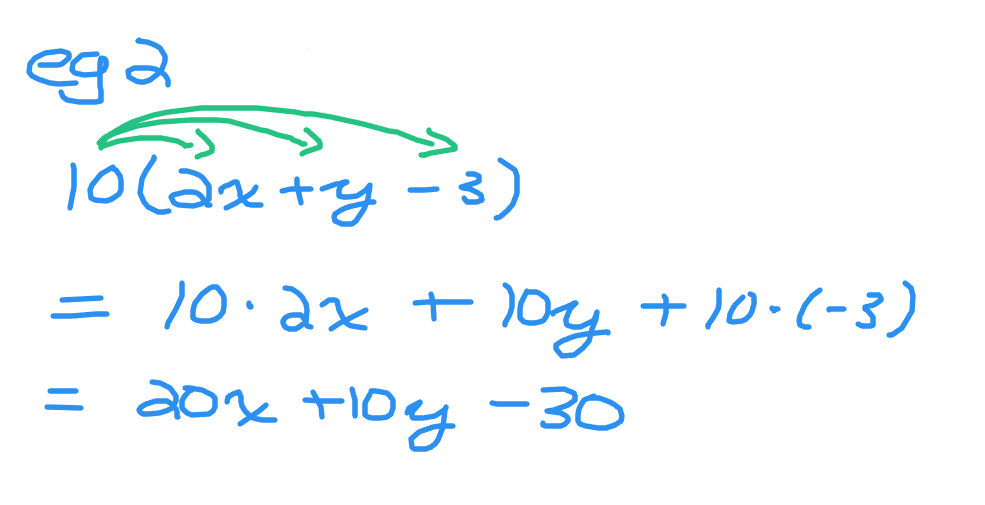
![example02.PNG](https://images.hive.blog/DQmYUQ9BqZKgPZpjZmTDoUttLxVznYuKQwYZoZA1bDfBZqd/example02.PNG)
</center>


&nbsp;

**Example Three**

What is `-2(x - 3y)`?

First multiply negative 2 with x from inside the bracket. The second term would be negative two multiplied by negative 3y. Remember that negative multiplied by a negative gives a positive quantity. 

<center>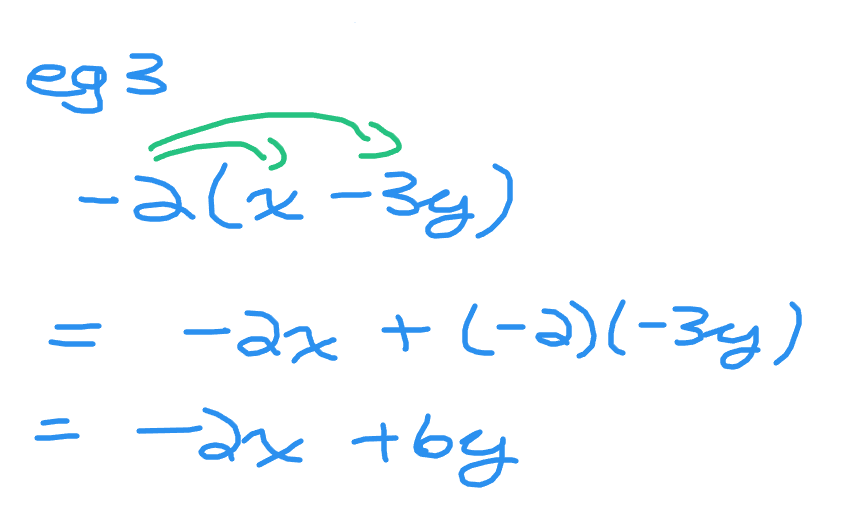
![example03.PNG](https://images.hive.blog/DQmPMSRzcjZuckwnsyVUHi5pZR7azTEU95eoNakDNPssNGQ/example03.PNG)
</center>

**Example Four**

Expand `-5(3x^2 - 8)`.

With this one be careful with multiplying negative numbers.

<center>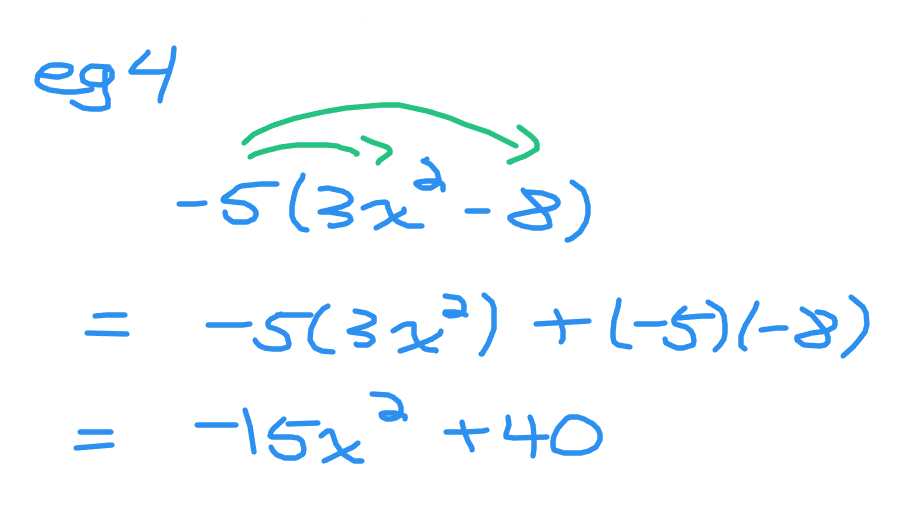
![example04.PNG](https://images.hive.blog/DQmRe56wqMnhRkGNsjAQPpba3CbNBryNaydHiPRXquXDxry/example04.PNG)
</center>

&nbsp;

**Example Five - Solving An Area Question**

The area of a rectangle is 60 square centimetres. Its length is 12 centimeters and the width measure is `x + 3` centimetres. What is the value of `x` and what is the width measure?

<center>
![example05.PNG](https://images.hive.blog/DQmVWxPrzGhsBDmSdVcf3M4tgRMPSrMqoeapa9zwLRPs7xw/example05.PNG)
</center>

&nbsp;

<center>Thank you for reading.</center>

Posted with [STEMGeeks](https://stemgeeks.net)